What Are Math Symbols?
Math symbols are concise marks, signs, or notations representing mathematical operations, quantities, relations, and functions. These symbols help to represent mathematical concepts and equations concisely.
Math symbols turn a lengthy explanation into a quick, easy calculation, helping you easily find the answer.
Example: Imagine you’re planning to find the area of a garden. Instead of writing “length times width” every time, math symbols let you simply jot down “l × w.” So, if your garden is 10 meters long and 4 meters wide, instead of saying “ten meters multiplied by four meters,” you can quickly see that 10 × 4 = 40 square meters.
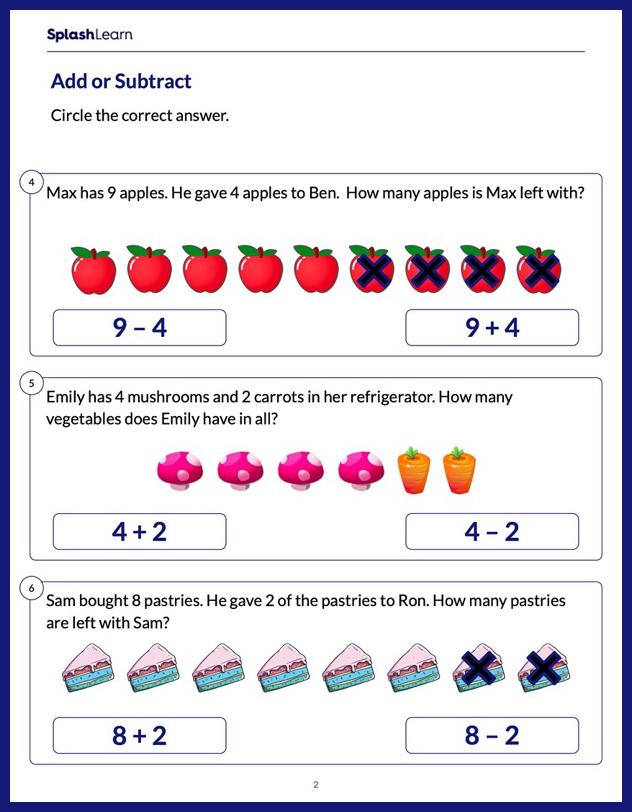
Symbols vary across different areas of mathematics, enabling precise communication of mathematical concepts and abstract ideas.
Recommended Games
Math Symbols for Arithmetic Operations
Arithmetic is a fundamental branch of mathematics focused on numbers and their relationships. It encompasses basic operations like addition, subtraction, multiplication, and division, which are integral to our daily activities.
| Symbols | Math Symbol Names | Meaning | Examples |
|---|---|---|---|
| $+$ | Plus sign | Addition | $1 + 2$ |
| $-$ | Minus sign | Subtraction | $3 – 2$ |
| $\times$ | Times sign | Multiplication | $5 \times 8$ |
| $\div$ | Division sign (obelus) | Division | $9 \div 3$ |
Recommended Worksheets
Commonly Used Mathematical Symbols
The mathematics vocabulary is rich in a wide variety of symbols. Compiling all math symbols here is not possible, but the table below shows the list of math symbols that are commonly used, their names, definitions, and examples.
| Symbols | Meaning | Examples |
|---|---|---|
| $=$ | Equal to sign (Is equal to) | $1 + 2 = 3$ |
| $\neq$ | Not equal to | $5 + 4 \neq 2$ |
| $\pm$ | Both plus and minus | $3(x \pm y) = 3x \pm 3y$ |
| Sometimes used to indicate a range | $3 \pm 1$ means numbers from 2 to 4. | |
| $\mp$ | Both minus and plus | $-\;2(a \pm b)= -\;2a \mp 2b$ |
| $\equiv$ | Identical to | $(a + b)^{2} ≡ a^{2} + 2ab + b^{2}$ |
| $\approx$ | Almost equal to (approximately equal to) | $\pi \approx 3.14$ |
| $\neq$ | Not equal to | $5 + 4 \neq 2$ |
| $.$ | Multiplication dot | $(5).(4) = 20$ |
| $/$ | Division slash | $8 \div 2 = 4$ |
| $\lt$ | Strictly less than | $5 \lt 10$ |
| $\gt$ | Strictly greater than | $10 \gt 5$ |
| $\le$ | Less than or equal to | $x \le 3$ |
| $\ge$ | Greater than or equal to | $x \ge 3$ |
| $\ll$ | Much less than | $0.001 \ll 1,000,000$ |
| $\gg$ | Much greater than | $1000 gg y$ |
| $\%$ | Percentage | $\frac{50}{100} = 50\%$ |
| $.$ | Period, decimal point | $0.75$ |
| $\overline{}$ | Vinculum (the line separating the numerator & denominator) | $\frac{1}{2}$ |
| $\propto$ | Proportional to | $x \propto y$ |
| $\ln$ | Natural logarithm (log to the base e) | $\ln 1 = log_{e} 1 = 0$ |
| $\sqrt{}$ | Square root | $\sqrt{4} = 2$ |
| $3\sqrt{}$ | Cube root | $3\sqrt{27} = 3$ |
| $n\sqrt{}$ | nth root | $5\sqrt{32} = 2$ |
| $\left( \right)$ | Parentheses (Round brackets) | $(a + b)^{2}$ |
| $\left[ \right]$ | Square brackets (brackets) | $\left[a-(b+3)\right]^{2}$ |
| $\left\{ \right\}$ | Curly brackets (Braces) | $\left\{[a-(b+3)]^{2}\right\}^{3}$ |
| $\in$ | Belongs to | $x \in A$ |
| $\notin$ | Does not belong to | $x \notin A$ |
| $\therefore$ | Therefore | $\therefore x = 1$ |
| $\because$ | Because | $b = 2 \because b = a$ and $a = 2$ |
| $\infty$ | Infinity | $x \rightarrow \infty$ |
| $\Sigma$ | Sigma (summation of all values in the given range) | $^{3}\Sigma_{i=1}\; i = 1 + 2 + 3 = 6$ |
Geometry Symbols
Geometry is a branch of mathematics that deals with the properties and relationships of points, lines, angles, surfaces, and solids. Understanding concepts such as length, width, area, volume, and perimeter is essential for solving geometric problems.
Symbols often represent specific terms in geometry to facilitate easier communication and problem-solving. These symbols are shorthand for complex geometric terms, making calculations and explanations more efficient. In daily life, these geometric symbols are ubiquitous, helping to quantify and describe the world around us in terms of shape, size, and position.
The table below lists the most commonly used geometric symbols, accompanied by examples to illustrate their application.
| Symbols | Meaning | Examples |
|---|---|---|
| $\angle$ | angle | $∠XYZ$ |
| $\measuredangle$ | measured angle | $\measuredangle\;LMN = 45^{\circ}$ |
| $\triangle$ | triangle | $\triangle\;DEF$ |
| $\cong$ | Congruent to | $\triangle\; PQR \cong \triangle \;ABC$ |
| $\sim$ | Similar to | $\triangle \; PQR \sim \triangle \;ABC$ |
| $\perp$ | is perpendicular to | $CD \perp XY$ |
| $∟$ | right angle | $∟\;A$ |
| $\parallel$ | is parallel to | $AB \parallel CD$ |
| $\circ$ | Degree | $50^{\circ}$ |
| (x, y) | Ordered pair (represents coordinates of a point) | (1, 2) |
| $\overline{AB}$ | Line segment | Line segment CD |
| $\overleftrightarrow{AB}$ | Line | Line AB |
| $\overrightarrow{AB}$ | Ray | Ray AB |
Math Symbols in Set Theory, Probability, and Combinatorics
| Symbol | Meaning | Formula | Example |
|---|---|---|---|
| ! | Factorial | n!= n.(n-1).(n-2)…3.2.1 | 3! = 3.2.1 = 6 |
| nPr | Number of permutations of n distinct objects, taken r at a time | nPr $= \frac{n!}{(n-r)!}$ | 3P2 $= \frac{3!}{(3-2)!} = 6$ |
| nCr | Number of combinations of n distinct objects, taken r at a time | nCr $= \frac{n!}{(n-r)!}$ | 3C2 $= \frac{3!}{(3-2)!2!} = 3$ |
| P( ) | Probability of an event | P(A) $= \frac{n(A)}{n(S)}$ | P(B) $= 1$ |
| {} | Empty set | – | A $= \left\{\right\}$ |
| ∅ | Empty set | – | B = ∅ |
| $\cup$ | Union of sets | $A \cup B = \left\{\text{x | x is in A or B}\right\}$ | $A \cup B$ |
| $\cap$ | Intersection of sets | $A\cap B = \left\{\text{x | x is in A and B}\right\}$ | $A\cap B$ |
Mathematical Constants Used as Math Symbols
In mathematics, ‘constants’ refer to entities that maintain fixed values, unlike variables that can change. Constants can encompass essential numbers, fundamental sets, specific concepts of infinity, and pivotal mathematical objects like the identity matrix. Often represented by letters of the alphabet or their variations, these constants are integral to mathematical expressions and equations. Below is a curated table detailing the most frequently encountered constants, including their names, definitions, and applications.
| Symbol | Description | Value |
|---|---|---|
| e | Euler’s constant; the base of the natural logarithm | e ≈ 2.71828 |
| π | Pi: The ratio of a circle’s circumference to its diameter | π ≈ 3.14159 |
| i | The square root of -1 | $i = \sqrt{-\;1}$ |
| φ | Golden ratio | φ ≈ 1.61803 |
| $\sqrt{2}$ | Pythagoras’ Constant | $\sqrt{2}$ ≈ 1.41421 |
Math Symbols Used in Logic (Boolean Algebra)
| Symbols | Meaning | Examples |
|---|---|---|
| ^ | and | p ^ q |
| ∨ | or | p ∨ q |
| ∃ | there exists | ∃ x |
| ∀ | for all | n2 > 1 ∀ n > 1 |
| ⟹ | implies | x = 2 ⟹ x2 = 4 |
| ⟺ | if and only if | x + 1 = y + 1 ⟺ x = y |
| | or : | such that | A = { x | x is a natural number. } A = {1, 2, 3,…} |
Numeric Symbols in Different Number Systems
Numbers serve as abstract symbols to denote arithmetic values, enabling us to count, measure, and categorize objects. When dealing with large quantities, such as 4,657, verbal descriptions become cumbersome. To address this, numerical symbols are employed for more efficient communication. The table below presents these quantities alongside their equivalents in Hindu-Arabic and Roman numeral systems, illustrating the diverse ways we represent numbers.
| Number Name | Hindu-Arabic Numerals | Roman numeral | Binary |
|---|---|---|---|
| Zero | 0 | Did not exist | 0000 |
| One | 1 | I | 0001 |
| Two | 2 | II | 0010 |
| Three | 3 | III | 0011 |
| Four | 4 | IV | 0100 |
| Five | 5 | V | 0101 |
| Six | 6 | VI | 0110 |
| Seven | 7 | VII | 0111 |
| Eight | 8 | VIII | 1000 |
| Nine | 9 | IX | 1001 |
| Ten | 10 | X | 1010 |
| Eleven | 11 | XI | 1011 |
| Twelve | 12 | XII | 1100 |
| Thirteen | 13 | XIII | 1101 |
| Fourteen | 14 | XIV | 1110 |
| Fifteen | 15 | XV | 1111 |
| Sixteen | 16 | XVI | 10000 |
| Seventeen | 17 | XVII | 10001 |
| Eighteen | 18 | XVIII | 10010 |
| Nineteen | 19 | XIX | 10011 |
| Twenty | 20 | XX | 10100 |
| Thirty | 30 | XXX | 11110 |
| Forty | 40 | XL | 101000 |
| Fifty | 50 | L | 110010 |
| Sixty | 60 | LX | 111100 |
| Seventy | 70 | LXX | 1000110 |
| Eighty | 80 | LXXX | 1010000 |
| Ninety | 90 | XC | 1011010 |
| Hundred | 100 | C | 1100100 |
Importance of Mathematical Symbols
Mathematical symbols serve as a foundational tool in mathematics, streamlining communication and understanding across various aspects of the discipline. Here’s an organized overview of their significance:
- Simplifying Language: Mathematical symbols streamline the expression of relationships and statements.
- Denoting Quantities: They provide a concise way to represent numerical values.
- Facilitating Discussion: Symbols ease the referencing and analysis of mathematical concepts.
- Clarifying Operations: They specify the operations to be performed, reducing errors.
- Universal Communication: Symbols transcend language barriers, enabling global understanding.
Facts about Math Symbols
- A Scottish mathematician, Robert Recorde, invented the “equal to sign” in 1557.
- The common symbol for infinity, ∞, was invented by the English mathematician John Wallis in 1655.
- In mathematics, an exclamation mark (!) represents a factorial symbol.
Conclusion
In this article, we learned about the different math symbols and their crucial role in simplifying and conveying complex mathematical concepts. These symbols form the backbone of mathematical communication, enabling precise and efficient problem-solving. To further our understanding, let’s solve a few examples and practice MCQs on these symbols for better comprehension.
Solved Examples on Math Symbols
1. Express using different math symbols:
i) the sum of a and b
ii) eight times y
iii) x to the power of 5
Solution:
i) The expression “sum of a and b” refers to the addition of the terms “a” and “b.”
Sum of a and b $= a + b$
ii) eight times y
Eight $= 8$
Here, y is a variable.
“Eight times x” refers to the multiplication of 8 and x.
Eight times $y = 8 \times y = 8y$
We can skip the multiplication symbol when we multiply a constant and a variable.
iii) x to the power of 5 means the base is x and the exponent is 5
x to the power of $5 = x^{5}$
2. Identify the meanings of the math symbols.
i) %
ii) $\triangle$
iii) ( )
iv) <
v) e
Solution:
i) % – Percentage
ii) $\triangle$ Triangle
iii) ( ) – Parentheses OR round brackets
iv) < – strictly less than
v) e – The e symbol in math represents the Euler’s constant.
3. Interpret the meanings using the math symbols.
i) $3 + 6$
ii) $x \neq 1$
iii) $a \parallel b$
Solution:
| Expression | Meaning |
|---|---|
| $3 + 6 \lt 10$ | The sum of 3 and 6 is strictly less than 10. |
| $x \neq 1$ | The value of the variable x is not equal to 1. |
| $a \parallel b$ | The line a is parallel to the line b. |
4. What are and-or symbols in logic? Give simple examples.
Solution:
In mathematics and logic, the “and” symbol is represented by ∧ and the “or” symbol by ∨. Here’s a simple example using these symbols:
Example 1:
Statement: “It is raining and cold.”
Mathematical Expression: Let statement p represent “It is raining” and q represent “It is cold.” The expression becomes p∧q.
Example 2:
Statement: “You can have tea or coffee.”
Mathematical Expression: Let statement a represent “You can have tea” and
b represent “You can have coffee.” The expression becomes a∨b.
Practice Problems on Math Symbols
Math Symbols - Definition with Examples
Using math symbols, how can you represent "p divided by q"?
There are different division symbols.
p divided by q can be written as $p \div q$ or $p/q$ or $\frac{p}{q}$.
If $l \bot m$, it means that
The statement $l \bot m$ means that the lines l and m are perpendicular to each other.
How is "the square root of number k" symbolically expressed?
The square root of the number $k = \sqrt{k}$
How would you symbolically state that "variable r is greater than or equal to 10"?
Variable r is greater than or equal to $10: r \ge 10$
What is this symbol in math? $\propto$
The symbol $\propto$ is used to represent proportional relationships.
Frequently Asked Questions about Math Symbols
Is the infinity symbol a real number?
No, the infinity symbol (∞) represents an unbounded quantity, not a specific number, and is used in mathematics to describe potential infinity.
Who created the symbol for pi?
The symbol for pi, π, was created by British mathematician William Jones in 1706 and later popularized by the Swiss mathematician Leonhard Euler. The symbol is a Greek letter.
What’s the “divisible by” symbol?
A vertical bar is used to represent divisibility. Example: m|n means “m divides n” or “n is divisible by m.”