30 60 90 Triangle – Definition with Examples

A three sided polygon is known as a triangle. A triangle has 3 sides, 3 vertices and 3 angles. There are different ways in which we can categorize the triangles. On the basis of sides, a triangle can be scalene, isosceles, or equilateral. However, in some cases, a triangle may possess unique properties. Such triangles are called special triangles. One such special triangle is the 30°–60°–90° triangles.

What Is a “30-60-90” Triangle?

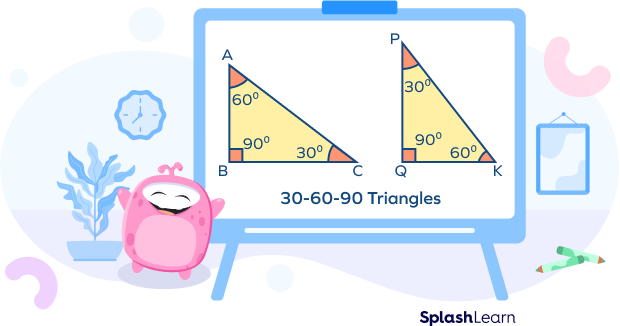
A special right triangle with angles 30°, 60°, and 90° is called a 30-60-90 triangle. The angles of a 30-60-90 triangle are in the ratio 1 : 2 : 3. Since 30° is the smallest angle in the triangle, the side opposite to the 30° angle is always the smallest (shortest leg). The side opposite to the 60° angle is the longer leg, and finally, the side opposite to the 90° angle is the largest side of the right triangle, also known as the hypotenuse.

Since the 30-60-90 triangle is a special triangle, the side lengths of the 30-60-90 triangle are in a constant relationship.

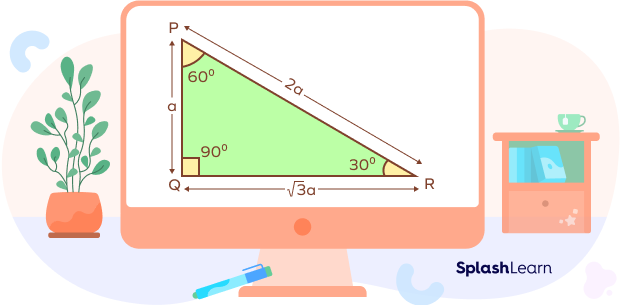
From the figure above, we can make the following observations about the side length ratio of a 30-60-90 triangle:

• The side opposite the 30° angle: PQ = a
• The side opposite the 60° angle: QR = a√3
• The side opposite the 90° angle: PR = 2a

The sides of a 30-60-90 triangle are always in the ratio of 1 : √3 : 2. 

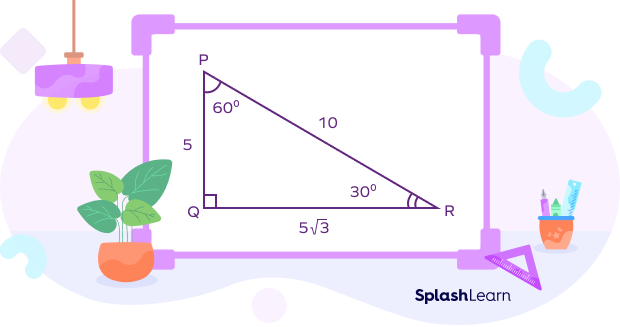
For example:

Here, in triangle PQR, 

• The side opposite to the 30° angle is PQ = a = 5 units 
• The side opposite to the 60° angle is QR = a√3 = 5√3 units
• The side opposite to the 90° angle is PQ = 2a = 10 units

30-60-90 Triangle Rule

In a 30-60-90 triangle, we can find the measure of any of the three sides by knowing the measure of at least one side in the triangle. This is known as the 30-60-90 triangle rule. The following shows how to find the sides of a 30-60-90 triangle using this rule: 

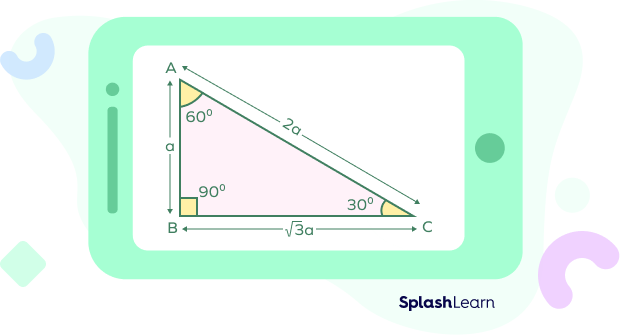
• When the side opposite to 30° is given.

Consider the side opposite to 30° (shortest side), AB to be ‘a’.

The side opposite to 60°, BC = a√3 

The side opposite to 90° (hypotenuse), AC = 2a

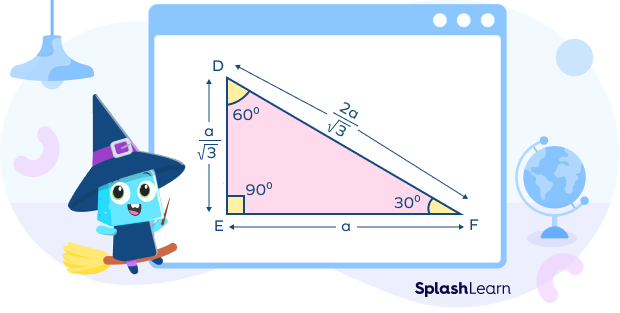
• When the side opposite to 60° is given.

Consider the side opposite to 60°, EF to be ‘a’.

The side opposite to 30°, DE = $\frac{a}{\sqrt{3}}$

The side opposite to 90° (hypotenuse), DF = $\frac{2a}{\sqrt{3}}$

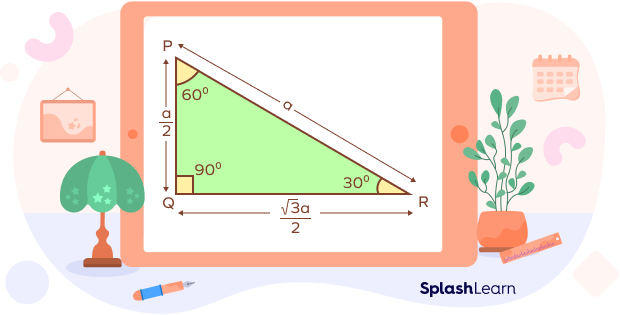
• When hypotenuse is given.

Consider the hypotenuse of the triangle, i.e., PR to be ‘a’.

The side opposite to 30°, PQ = $\frac{a}{2}$

The side opposite to 60°, QR = $\frac{\sqrt{3}}{2}$a

Area of the 30-60-90 Triangle

When the perpendicular is given to be a, 

Area of $\Delta$DEF = $\frac{1}{2} \times base \times height$

= $\frac{1}{2} \times a \sqrt{3} \times a = \frac{\sqrt{3}a^{2}}{2}$

Conclusion

In this article, we learned about a special triangle, i.e., the 30-60-90 triangle. To read more such informative articles on other concepts, visit our website. We, at SplashLearn, are on a mission to make learning fun and interactive for all students.

Solved Examples

1. Find the length of the side BC in the following figure.

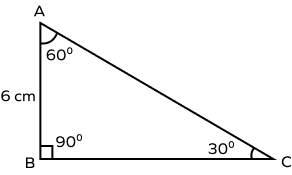
Answer: The side opposite to 30˚ (shortest side), AB = 6 cm

Since the sides of a 30-60-90 triangle are always in the ratio of 1 : √3 : 2. 

Therefore, the sides of the given triangle are AB = 6 cm, BC = 6√3 cm and AC = 12 cm. 

So, BC = 6√3 cm

2. Find the length of the hypotenuse in the following figure.

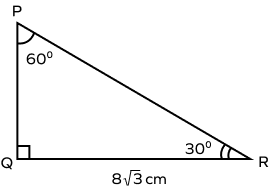
Answer: Side opposite to 60˚, QR = a = 8√3 cm 

The hypotenuse of the triangle, i.e., PR = $\frac{2a}{\sqrt{3}} = \frac{2 × 8√3}{\sqrt{3}}$ = 16 units. 

3.  A triangle has sides 3√2, 3√6, and 3√8. Find the angles of this triangle.

Answer: The sides of the triangle are 3√2, 3√6, and 3√8.

Let us check whether the sides are of the 30-60-90 triangle. 

On dividing each side of the triangle by 3√2, we get 1, √3, and 2. Therefore, sides of the triangle are 3√2, 3√6, and 3√8, are in the ratio 1: √3: 2.

The sides are following the 30-60-90 triangle rule. So, the angles of the given triangle are 30°, 60°, and 90°.

Practice Problems

Frequently Asked Questions