Volume of a Sphere

Volume of a Sphere – Introduction

Have you ever wondered, “I can draw a circle, but I cannot draw a sphere? Why?” This is because a circle is a two-dimensional figure and does not have volume, whereas a sphere is a three-dimensional shape with no edges or vertices. That means its points lie in space. Hence, you cannot draw it. This is the reason we always find the volume of a sphere to calculate the amount of space it occupies. 

Scroll ahead to learn about the volume of a sphere formula, the derivation of the sphere volume formula, some solved examples, facts, and more.

What Is the Volume of a Sphere?

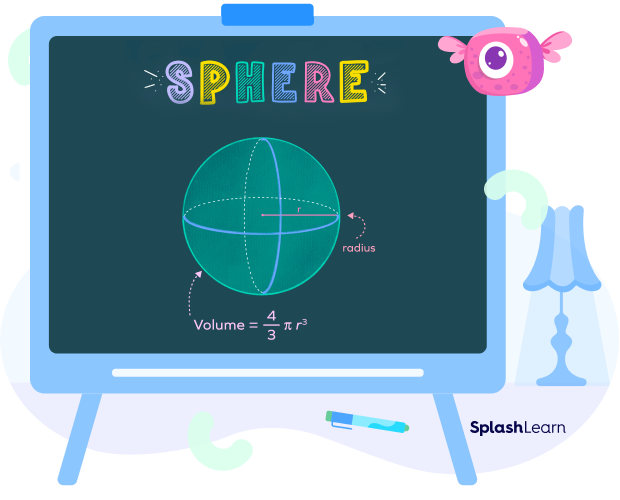
Wondering how we can find the volume of a sphere? Hold on, we will get to that, but first, understand what the volume of a sphere means. The volume of a sphere is the measure of three-dimensional space occupied by a sphere. It depends on the sphere’s radius, which is half the diameter (the longest line inside the sphere that passes through the center of the sphere). 

That means if the radius of the sphere changes, its volume changes too!

The volume of a sphere is measured in cubic units, such as $m^3$, $cm^3$, and so on.

What Is the Formula to Find the Volume of a Sphere?

How do you find the volume of a sphere given that “r” is the radius of the sphere? The volume of a sphere equation is as follows:

The volume of a sphere $= \frac{4}{3} \pi r^3$

How to Calculate the Volume of a Sphere?

Suppose the radius of the sphere is 6 cm, then its volume will be:

As we know, the volume of the sphere, V $= \frac{4}{3} \pi r^3$

Here, $r = 6$ cm

Thus, volume of sphere, V $= \frac{4}{3} \pi r^3 = \frac{4}{3} \times 3.14 \times 6 \times 6 \times 6$ 

$\Rightarrow$ V $= 904.32 cm^3$

Therefore, the volume of a sphere is 904.32 cm3

Volume of Solid Sphere

If the radius of the solid sphere is r, the volume of the sphere is given by:

Volume of Sphere, V $= \frac{4}{3} \pi r^3$

Volume of Hollow Sphere

Sphere that has a cavity or space inside is called a hollow sphere. 

Let the radius of the outer sphere is R and the radius of the inner sphere is r. 

The volume of the sphere, V is given by:

Volume of Sphere, V = Volume of Outer Sphere – Volume of Inner Sphere 

$= \frac{4}{3} \pi R^3 – \frac{4}{3} r^3$

$= \frac{4}{3} \pi (R^3 – r^3)$

Fun Facts about Spheres

How about some fun and interesting facts about the sphere? Let’s take a look!

1. A sphere is symmetrical and round. It does not have any faces, corners, or edges.
2. Balls, marbles, and even Earth are shaped like spheres.
3. A hemisphere is an exact half of a sphere.
4. All the sphere’s surface points are the same distance “r” from the center.
5. The sphere appears in nature when a surface wants to be as small as possible. For example, if you blow up a balloon, it naturally forms a sphere!

Conclusion

So, how was the lesson? We hope you have got a clear understanding of the volume of spheres. Stay tuned with SplashLearn for more useful math lessons like these.

Solved Examples

1. What is the volume of a sphere with a radius of 12 units?

Solution: To solve for the volume of a sphere, you must first know the equation for the volume of a sphere.

The equation for the volume of a sphere is:

V $= \frac{4}{3} \pi r^3$

If the radius of the sphere is 12, then you plug that in for r and solve:

V $= \frac{4}{3} \pi r^3 = \frac{4}{3} \times 3.14 \times 12 \times 12 \times 12 = 7234.56$ cubic units 

2. Find the volume of the sphere whose diameter is 28 cm.

Solution: Given, diameter $= 28 cm$

So, radius $= \frac{Diameter}{2} = \frac{28}{2} = 14 cm$

From there, we can use the formula, which is

V $= \frac{4}{3} \pi r^3 = \frac{4}{3} \times 3.14 \times 14 \times 14 \times 14 = 11488.23 cm^3$

3. Find the volume of a spherical tank whose radius is 3 inches.

Solution:

The equation for the volume of a sphere is:

V $= \frac{4}{3} \pi r^3$

If the radius of the sphere is 3 inches, then you plug that in for r and solve:

V $= \frac{4}{3} \pi r^3 = \frac{4}{3} \times 3.14 \times 3 \times 3 \times 3 = 113.04$ cubic inches 

4. Nina created a spherical ball of radius 5 cm using clay. She then cut the sphere into two equal parts. What will be the volume of each part?

Solution:

The equation for the volume of a sphere is:

V $= \frac{4}{3} \pi r^3$

If the radius of the sphere is 5 cm, then you plug that in for r and solve:

V $= \frac{4}{3} \pi r^3 = 43 3.14 5 5 5 = 523.33$ $cm^3$

Hence volume of each cut part $= \frac{523.33}{2} = 261.66$ $cm^3$

5.  Sam wants to create a sphere of radius 7 cm using some clay. What will be the volume of the sphere formed?

Solution:

The equation for the volume of a sphere is:

V $= \frac{4}{3} \pi r^3$

If the radius of the sphere is 7 cm, then you plug that in for r and solve:

V $= \frac{4}{3} \pi r^3 = \frac{4}{3} \times 3.14 \times 7 \times 7 \times 7 = 1436.02$ $cm^3$

Practice Problems

Frequently Asked Questions

Related Articles

• Volume
• Cone
• Sphere