What Is Reflexive Property?
Reflexive property is a fundamental property of relations which states that every element of a set is related to itself by that relation. Mathematically, a relation R on a set A is said to be reflexive if for all a ∈ A, (a, a) ∈ R.
You can think of the reflexive property as finding a reflection of yourself in a mirror. The reflexive property on a set states that every component/element is related to itself. In the case of congruence, it is known as the reflexive property of congruence. If the case is of equality, it is known as the reflexive property of equality.
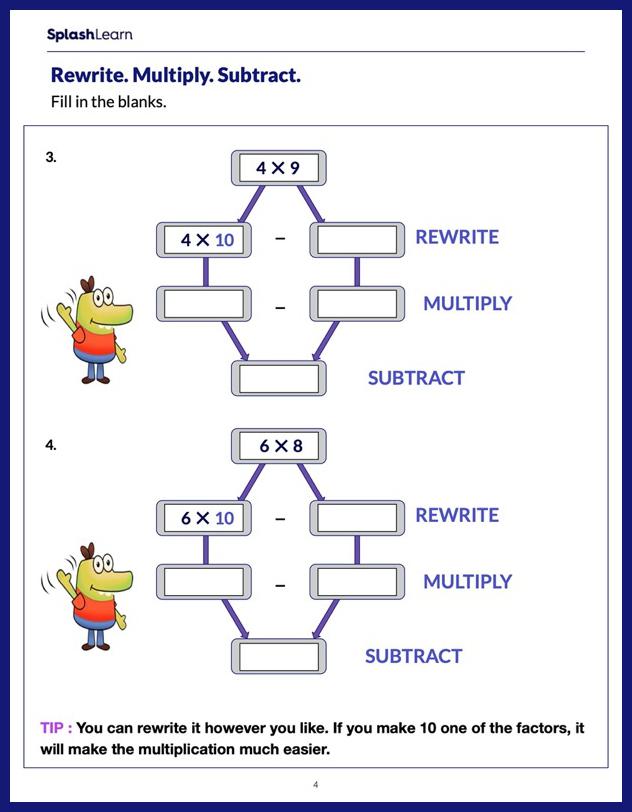
Recommended Games
Reflexive Property of Congruence
The reflexive property of congruence states that every geometric shape or geometric figure is congruent to itself.
When two shapes have the same size and shape, they are said to be congruent. The symbol for congruence is ≅. Reflexive property in geometry is pretty easy to understand.
Reflexive property of congruence examples:
By the reflexive property of segment congruence, we can say that every line segment is congruent to itself.
Recommended Worksheets
Reflexive Property of Equality
The reflexive property of equality states that every number is equal to itself. For any number a, we have $a = a$.
Examples:
$1 = 1$
$\;-\;7 = \;-\;7$
$x = x$
Define the relation of equality on the set of real numbers as R such that a R b if and only if a = b.
The relation R is an equivalence relation since it is reflexive, symmetric, and transitive.
i) Reflexive property: $a = a$
ii) Symmetric property: If $a = b$, then $b = a$.
iii) Transitive property: If $a = b$ and $b = c$, then $a = c$.
Reflexive Property of Relations
A binary relation R defined on a set A is said to be reflexive if, for every element a ∈ A, we have aRa, that is, (a, a) ∈ R. If R is a reflexive relation on a set A, then every element of A is related to itself by R.
An example of the relation “is equal to.” It is reflexive as every number is equal to itself. Also, the reflexive property of congruence and equality are ultimate cases of reflexive property of relations. An example of the reflexive property of equality is “is a subset of,” as we know, every set is a subset of itself.
Example: Is the relation $R = \left\{ (1,\; 1),\; (2,\; 2),\; (1,\; 2) \right\}$ defined on the set $\left\{1, 2\right\}$ reflexive?
1 R 1 since (1, 1) ∈ R
2 R 2 since (2, 2) ∈ R
Each element in the set is related to itself. Thus, the relation is reflexive.
Facts about Reflexive Property
- In mathematical terms, the reflexive property simply states that $a = a$ where “a” is a real number.
- We can find applications of the reflexive property in different mathematical proofs and operations.
Conclusion
In this article, we learned about the reflexive property, reflexive property of equality, and reflexive property of congruence. Let’s solve a few examples and practice problems.
Solved Examples of Reflexive Property
1. Is the relation $R = \left\{ (a,\; a),\; (a,\; b),\; (b,\; b) \right\}$ defined on the set $A = \left\{a,\; b,\; c\right\}$ reflexive?
Solution:
$A = \left\{a,\; b,\; c\right\}$
$R = \left\{ (a,\; a),\; (a,\; b),\; (b,\; b) \right\}$
a R a since (a, a) ∈ R
b R b since (b, b) ∈ R
(c, c) R
Thus, the element c is not related to itself.
The relation R is not reflexive.
2. A binary relation R is defined on the set of real numbers. For natural numbers a and b, we have a R b if and only if $a \lt b$. Is the relation reflexive?
Solution:
A relation is said to be reflexive if every element of the set is related to itself.
The “less than $(\lt)$” relation is not reflexive because a number can never be less than itself.
For any real number, the statement $a \lt a$ is not true.
Thus, R is not reflexive.
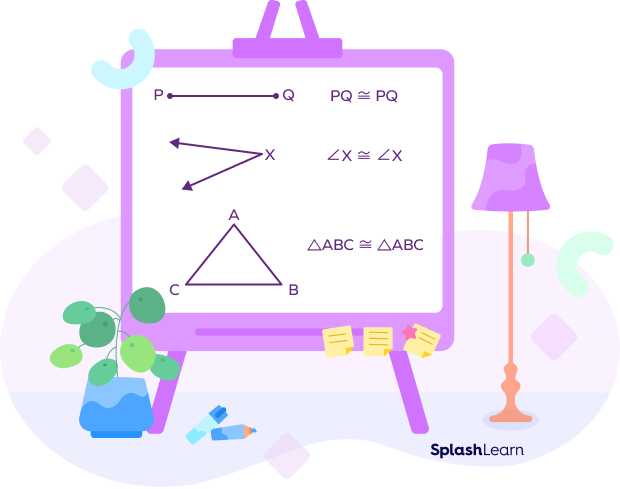
3. What does the reflexive property of congruence state for a line segment PQ, an angle X, and a triangle ABC.
Solution:
Reflexive property of congruence states that every geometric figure is congruent to itself.
PQ ≅ PQ
∠X ≅ ∠X
ABC ≅ ABC
4. Observe the diagram below and write statements based on the reflexive property.

Solution:
Every triangle is congruent to itself.
AOB ≅ AOB
POQ ≅ POQ
Every line segment is congruent to itself.
OA ≅ OA, OB ≅ OB, AB ≅ AB
OP ≅ OP, OQ ≅ OQ, PQ ≅ PQ
Every angle is congruent to itself.
∠A ≅ ∠A, ∠B ≅ ∠B,∠O ≅ ∠O, ∠P ≅ ∠P, ∠Q ≅ ∠Q
Practice Problems on Reflexive Property
Reflexive Property - Definition, Equality, Examples, FAQs
Which statement is NOT based on the reflexive property of equality?
Reflexive property of equality for real numbers states that $a = a$, for any real number a.
The statement $a = \;-\;8$ simply states that the value of the variable a is $\;-\;8$. It is not based on the reflexive property of equality.
For any real number r, the statement $r = r$ is based on the
By the reflexive property of equality, every real number “r” is equal to itself.
It can be denoted as$ r = r$.
Which of the following expressions is NOT based on the reflexive property of congruence?
By the reflexive property of congruence, every geometric figure (an angle, line segment, or shape) is congruent to itself.
$| (AB) = | (BA)$ simply means that the length of the line segment remains the same. This is true regardless of the order of endpoints you choose to help name the segment. It is not based on the reflexive property of congruence.
Frequently Asked Questions about Reflexive Property
What is the equivalence relation?
A relation is an equivalence relation if it is reflexive, symmetric, and transitive.
Can the reflexive property be violated?
Yes, the reflexive property is violated in some conditions. For instance, in certain non-classical logic, there is a possibility for an object to not have a relation to itself. This is called non-reflexivity.
Is the “greater than or equal to” relation reflexive on the set of real numbers?
Yes, the “greater than or equal to” relation is reflexive since each real number is always equal to itself. For any real number x, we have $x \ge x$.
What is the transitive property of equality?
The transitive property states that for all real numbers a, b, and c
if $a = b$ and $b = c$, then $a = c$.
What is a binary relation?
A binary relation R between sets A and B is a subset of the cartesian product $A \times B$. It is a new set of ordered pairs (a, b) consisting of elements a in A and b in B.