Algebra – Definition, Examples, Practice Problems, FAQs

Algebra is the part of mathematics that helps represent problems or situations in the form of mathematical expressions.  In algebra, we use numbers like 2, −7, 0.068 etc., which have a definite or fixed value. In algebra we use variables like x, y, and z along with numbers.

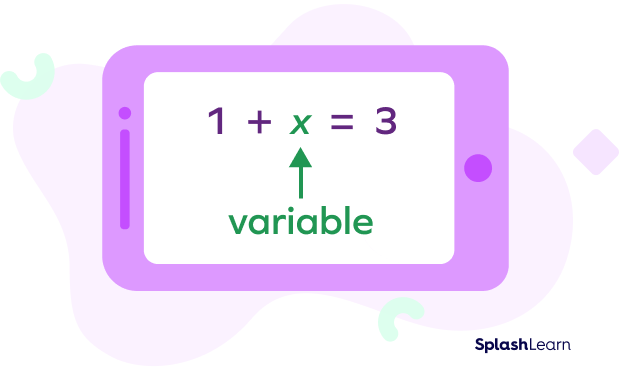
Signs used in algebra 

Mathematical operations like multiplication (×), division (÷), addition (+), and subtraction (−) are used to form a meaningful mathematical expression.

There are other signs and symbols that are also frequently used in algebra.

equal to (=), not equal to (≠), less than (<), greater than (＞), less than equal to(≤), greater than equal to ( ≥).

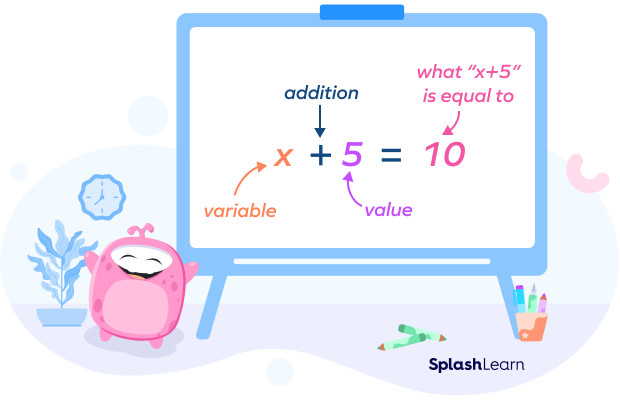
A straightforward example of an equation in algebra is x + 5 = 10.

Algebra A Puzzle

Algebra is all about puzzles. 

Here is one for you. 

 ⬜+ 10 = 13

⬜ plus 10 is equal to 13.

We have to find the number in this box, and what number, when added to 10, gives us 13. Simple, it’s three; we can see that 3 plus 10 equals 13. The puzzle we see here contains only numbers. 

We can also say that x plus 10 equals 13. Find x.

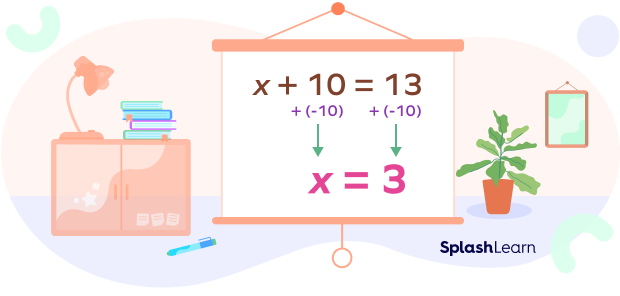
We use the letter in place of the box. These letters are also called variables, sometimes referred to as the unknown since their values are unknown to us.

Related Games

Evaluate Algebraic Expressions with One Operation Game

Evaluate Algebraic Expressions with Two Operations Game

Form Algebraic Expressions Game

Writing the Statements in Algebraic Form.

1. The total cost of 1 kg apple and 2 kg of guava is $3. If x represents the cost of 1 kg apple and y  represents the cost of 1 kg guava, then this statement is represented in the form of an algebraic expression as:

x + 2y = 3

2. If the area of the rectangle is 36 cm². Its length is represented as l  and width is represented as b. Then this statement is represented as :

l  × b = 36

Solved Examples on Algebra

Question 1: William had a few chocolates with him. Jack came across and took away five of his chocolates. And then he had just seven chocolates remaining with him. How many chocolates did he have before Jack came to him? 

Answer: Assume William had x chocolates with him, and Jack took away five of his chocolates.

x − 5 = 7

So, we subtract five from x. Moreover, after all this, William was left with seven chocolates. This entire puzzle will then equal seven. 

x − 5 + 5 = 7 + 5

x = 12

Question 2: A magician was invited to perform at a birthday party. The magician arrived at the party with 18 balloons. On the way, 7 balloons got blown away. So how many balloons did the magician have in the beginning?

Answer: We can solve this problem using algebra, x − 7 = 18.

So, we subtract seven from x, and after that, we are left with 18 balloons.

Now, all we have to do is find x.

x − 7 + 7= 18 + 7

x = 18 + 7

x = 25

Question 3: Amman wants to visit a zoo. A ticket for the entry to the zoo cost $4. He has only $3. How much more money does he need to buy the ticket?

Answer: Let Amman need $y more to buy the ticket.

So, we will solve this problem using algebra, 3 + y = 4

We add y to 3 to get 4.

Now we need to find the value of y.

3 + y − 3 = 4 − 3

y = 4 − 3

y = 1

Practice Problems on Algebra

Frequently Asked Questions on Algebra